Step Traversing a Tree

Memory limit: 32 MB

A graph is a pair , where is a finite set of elements called vertices of the graph, and is a subset of the set of all unordered pairs of distinct vertices. The elements of the set are called edges of the graph. If for each pair of distinct vertices , there exists exactly one sequence of distinct vertices , such that , and the pairs for , then the graph is called a tree. We say that the distance between the vertices and in the tree is .

It is known that a tree of vertices has exactly edges. A tree whose vertices are numbered from to can be unambiguously described by giving the number of its vertices , and an appropriate sequence of pairs of positive integers describing its edges.

Any permutation of vertices - i.e. a sequence in which each vertex appears exactly once - is called a traversing order of a tree. If the distance of each two consecutive vertices in some order of the tree is at most , then we say that it is a traversing order of the tree with step .

It is known that for each tree its traversing order with step can be found.

Example

The picture shows a tree of 7 vertices. The vertices are represented by black dots, and edges by line segments joining the dots.

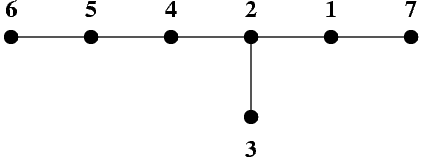
This tree can be traversed with step 3 by visiting its vertices in the following order: 7 2 3 5 6 4 1.

Task

Write a program that:

• reads a description of a tree from the standard input.
• finds an arbitrary traversing order of that tree with step ,
• writes that order in the standard output.

Input

• In the first line of the standard input there is a positive integer , not greater than - it is the number of vertices of the tree.
• In each of the following lines there is one pair of positive integers separated by a single space and representing one edge of the tree.

Output

In the successive lines of the standard output one should write the numbers of the successive vertices in a traversing order of the tree with step 3 - each number should be written in a separate line.

Example

For the input data:

7
1 2
2 3
2 4
4 5
5 6
1 7

the correct result is:

7
2
3
5
6
4
1

Task author: Krzysztof Diks.